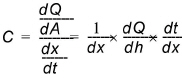
Courant number, C, represents the ratio of physical wave speed to the ‘grid speed’ and is calculated as
(24.16) 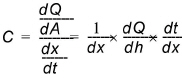
where dQ is the change in flow rate, dA is the change in cross-sectional area and dh is the change in water depth.
For a stable explicit solution the courant number must be less than one, which means that a wave cannot travel across a cell in less than one time step.
Solving for the time step, yields,
where Dt is the time step and Dx is the cell width.
The differential term in (24.17) is the inverse of the derivative of the Mannings equation, (24.13), with respect to h, which goes to zero as the change in water depth approaches zero. Thus, flat areas with flat water levels will require very small time steps. Likewise, smaller grid spacing will also lead to smaller time steps.